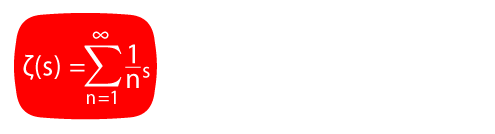A Mertens function analogue for the Rankin–Selberg L-function

Let f be a self-dual Maass form for $SL(n, Z)$. We write $L_f (s)$ for the Godement–Jacquet L-function associated to $f$ and $L_{f\times f} (s)$ for the Rankin–Selberg L-function of $f$ with itself. The inverse of $L_{f\times f} (s)$ is defined by
$$\frac{1}{L_{f\times f}(s)} := \sum_{m=1}^\infty \frac{c(m)}{m^s}, \mathfrak{R}(s) > 1.$$
It is well known that the classical Mertens function $M(x) := \sum_{m\leq x} \mu(m)$ is related to
$$\frac{1}{\zeta(s)} = \sum_{m=1}^\infty \frac{\mu(m)}{m^s}, \mathfrak{R}(s) > 1.$$
We define the analogue of the Mertens function for $L_{f\times f} (s)$ as $\widetilde{M}(x) := \sum_{m\leq x} c(m)$ and obtain an upper bound for this analogue $\widetilde{M}(x)$, similar to what is known for the Mertens function $M(x)$. In particular, we prove that $\widetilde{M}(x) \ll_f x \exp(−A\sqrt{\log{x}}$ for sufficiently large $x$ and for some positive constant $A$. This is a joint work with my Ph.D. supervisor Prof. A. Sankaranarayanan.