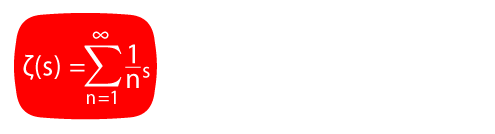One-level density of zeros of Dirichlet L-functions over function fields

We compute the one-level density of zeros of order-$\ell$ Dirichlet $L$-functions over function fields $\mathbb{F}_q[t]$ for $\ell=3,4$ in the Kummer setting ($q\equiv1\pmod{\ell}$) and for $\ell=3,4,6$ in the non-Kummer setting ($q\not\equiv1\pmod{\ell}$). In each case, we obtain a main term predicted by Random Matrix Theory (RMT) and a lower order term not predicted by RMT. We also confirm the symmetry type of the family is unitary, supporting the Katz and Sarnak philosophy.