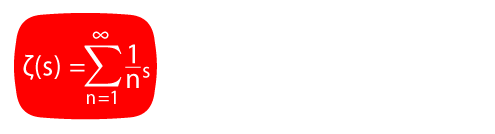Zeros of L-functions in low-lying intervals and de Branges spaces

We consider a variant of a problem first introduced by Hughes and Rudnick (2003) and generalized by Bernard (2015) concerning conditional bounds for small first zeros in a family of L-functions. Here we seek to estimate the size of the smallest intervals centered at a low-lying height for which we can guarantee the existence of a zero in a family of L-functions. This leads us to consider an extremal problem in analysis which we address by applying the framework of de Branges spaces, introduced in this context by Carneiro, Chirre, and Milinovich (2022).